Several entrants correctly answered the Balancing Act Geek Challenge. The correct answer is B, the scale tips to the right.
Correct answers were received from Tim Jager of DMC, Devon Fritz of DMC, Brandon Williams of Yaskawa, Ian Schleifer, and Gareth Meirion-Griffith of DMC.
Many people answered that the scales would tip to the left. The intuitive answer points that way. Since the pool ball is suspended externally, it is easy to attribute all of its weight to the external support. This would make the left side heaver by the weight of the wood ball. However, the intuitive assumption that the entire weight of the pool ball is supported externally is wrong. Because it is submerged, some of its weight is supported by buoyancy, and some is supported externally. The buoyant force transmits through the water to the scale. To determine which way the scale tips, these separated forces must be analyzed.
Of the correct answers received, Brandon Williams of Yaskawa answered the question most completely, and he is this month’s Geek Challenge winner. His solution is shown here:

The problem is easily solved by carefully selecting where to set the system boundaries. When the boundary is selected to completely encompass the ball and the water, the number of forces to resolve is immediately reduced.
Case 1 with the wooden ball simplifies to just the force of gravity because the buoyancy is canceled out inside the system. This means that there is no difference between whether the ball is in the water or not.
Case 2 simplifies to the force of gravity needing to balance out with the reaction force from the scale and the tension force of the rope. The tension of the rope can be easily solved by taking the weight of the ball and subtracting off the buoyant force. This means that the terms about the force of gravity of the ball can be canceled, and you are left with the force of gravity of the water and the buoyant force.
Since the force of gravity of the water cancels between the two sides, the problem is simply determining whether the buoyant force or the force of gravity of the wooden ball is greater. The buoyant force is defined by the force of gravity of an equivalent amount of water and the water is defined to have a higher density than the wood. Therefore, the equations will then make it clear that the buoyant force is greater.
This leads to the now obvious conclusion that the left side has less force and will therefore rise and the right side has more force and will descend.
Answer: B
Brandon’s logical proof is ironclad and mathematically produces the correct answer. Additionally, his inclusion of a nice Free Body Diagram wins the prize! Similar logic was used by many of the correct respondents listed above. I suspect, based on certainty expressed by many respondents convinced the system would tilt left, that this logical proof may not be enough to sway all parties. So I will offer an additional explanation as follows:
Assuming additionally that the two buckets are the exact same size, shape and weight, (an assumption not stated in the problem statement), the following system boundary makes the answer intuitive:
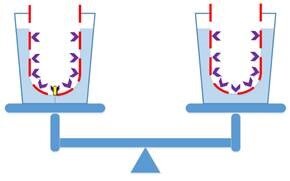
Both sides of the scale will see the weight of identical buckets, and the weight of an identical amount of water. Both scales will also see the force exerted by static water pressure at the boundary. The water pressure at any depth in an open-top container is proportional to the depth of the water, and the density of water. Since the volume of the balls was identical and both were completely submerged, the water level increase caused by the balls is identical. Therefore, the water height is identical, and the static water pressure at all points around the boundary is identical. Since I conveniently drew identical boundaries left and right, they have the same area and shape. The force of water at the boundary (pressure integrated over area) is equal.
What is not equal is a string attached to the left scale pulling up in tension. This force alone tips the scales to the right.
Submit your comments to geekchallenge@dmcinfo.com.